Hilbert transform pair
WebJul 22, 2011 · It is known that the poor translation-invariance of standard wavelet bases can be improved by considering a pair of wavelet bases, whose mother wavelets are related through the Hilbert transform [8, 7, 11, 4]. The advantages of using Hilbert wavelet pairs for signal analysis had also been recognized by other authors [1, 5]. The Hilbert transform is important in signal processing, where it is a component of the analytic representation of a real-valued signal u(t). The Hilbert transform was first introduced by David Hilbert in this setting, to solve a special case of the Riemann–Hilbert problem for analytic functions. See more In mathematics and signal processing, the Hilbert transform is a specific singular integral that takes a function, u(t) of a real variable and produces another function of a real variable H(u)(t). The Hilbert transform is given … See more The Hilbert transform arose in Hilbert's 1905 work on a problem Riemann posed concerning analytic functions, which has come to be known as the Riemann–Hilbert problem. Hilbert's work was mainly concerned with the Hilbert transform for functions defined on … See more It is by no means obvious that the Hilbert transform is well-defined at all, as the improper integral defining it must converge in a suitable sense. However, the Hilbert transform is well-defined for a broad class of functions, namely those in More precisely, if u … See more The Hilbert transform of u can be thought of as the convolution of u(t) with the function h(t) = 1/ π t, known as the Cauchy kernel. Because 1⁄t is not integrable across t = 0, the integral defining the convolution does not always converge. Instead, the Hilbert transform is … See more The Hilbert transform is a multiplier operator. The multiplier of H is σH(ω) = −i sgn(ω), where sgn is the signum function. Therefore: where See more In the following table, the frequency parameter $${\displaystyle \omega }$$ is real. Notes 1. ^ … See more Boundedness If 1 < p < ∞, then the Hilbert transform on $${\displaystyle L^{p}(\mathbb {R} )}$$ is a bounded linear operator, meaning that there exists a constant Cp such that for all $${\displaystyle u\in L^{p}(\mathbb {R} )}$$ See more
Hilbert transform pair
Did you know?
WebSep 1, 2013 · Hilbert transform pair with the usage of multipliers which increases the hardware complexity and cost. This drawback has been addressed in th is paper and an improved design is proposed. In this... WebThese two equations form a Hilbert transform pair. v(t) and u(t) are sometimes refered to as direct and inverse Hilbert transforms, respectively. Hilbert transforms are valid for the "principal value at x=t only" as denoted by the subscript P …
WebThe Hilbert transform is anti-self-adjoint. Therefore, it is natural to define it on distribution by passing H to the test functions, similar to "pass the hat" definition of the Fourier … WebAug 1, 2010 · The feasibility of Hartley–Hilbert transform for a straight forward interpretation, total magnetic anomaly due to a thin plate from Tejpur, India and self potential data of the Sulleymonkey anomaly in the Ergani Copper district, Turkey are illustrated in contrast with the Fourier–Hilbert transform. This pair of transforms have …
http://sepwww.stanford.edu/sep/prof/pvi/spec/paper_html/node2.html WebWe used a specific delay operator earlier to create the Hilbert transform in Chapter 13.Here we will comment on delay operators in general. Creation of a delay υ 1 in x (t) is an …
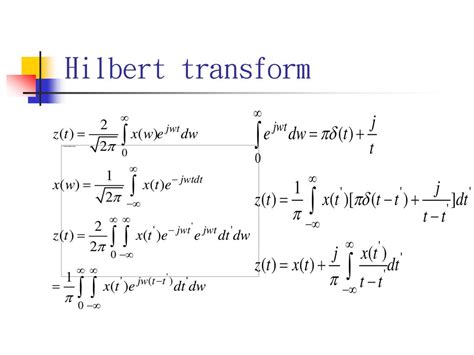
WebHilbert transform of a signal x (t) is defined as the transform in which phase angle of all components of the signal is shifted by ± 90 o. Hilbert transform of x (t) is represented with …
Webing and Hilbert-Huang decomposition. This work introduces a complex Hilbert transform (CHT) filter, where the real and imaginary parts are a Hilbert transform pair. The CHT filtered signal is analytic, i.e. its Fourier transform is zero in negative frequency range. The CHT filter is constructed by half-sample delay operators based on the B ... chirurgische praxis am filmparkWebLet x(t) have the Fourier transform X(ω). The Hilbert transform of x(t) will be denoted by ˆx(t) and its Fourier transform by Xˆ(ω). The Hilbert transform is defined by the integral xˆ(t) = x(t)∗ 1 πt = 1 π Z ∞ −∞ x(τ) t−τ dτ where ∗ represents convolution. Thus, the Hilbert transform of a signal is obtained by passing it chirurgische praxis bremen hornWebHILBERT TRANSFORM Chapter explains that many plots in this book have various interpretations. Superficially, the plot pairs represent cosine transforms of real even functions. But since the functions are even, their … graphisches abstractWebApr 11, 2024 · Download Citation Generalized spherical Aluthge transforms and binormality for commuting pairs of operators In this paper, we introduce the notion of generalized spherical Aluthge transforms ... graphisches symbol edvWebThe additional Hilbert Transform means that normal DWT implementations (eg., Daubechies's construction) won't work and filters need to be designed from scratch. Hilbert Transform condition Using dilation equations, the scaling function and wavelet for top branch (real) can be written as graphische stoffeWebWe use the fact that these components form a Hilbert transform pair to transform a skewed anomaly profile into a symmetric one. Unlike in previous works that rely on the decomposition into even and odd functions, the profile does not need to be shifted to the source's center of symmetry or limited to one isolated anomaly. Multiple effective ... graphische symbole medizinprodukteWeb3. The Hilbert transform is anti-self-adjoint. Therefore, it is natural to define it on distribution by passing H to the test functions, similar to "pass the hat" definition of the Fourier transform. In fact, the Wikipedia article already says this. Since the stated relation between F and H holds for test functions, the duality-based definition ... chirurgische praxis cottbus